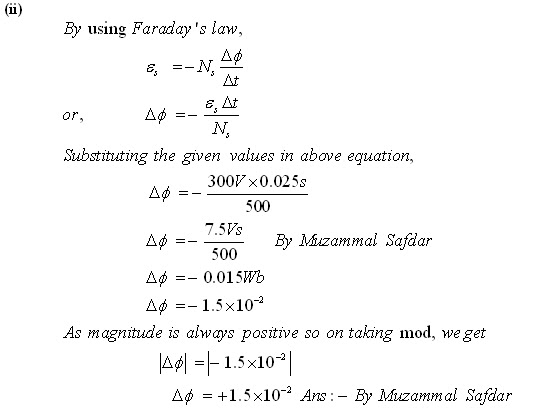
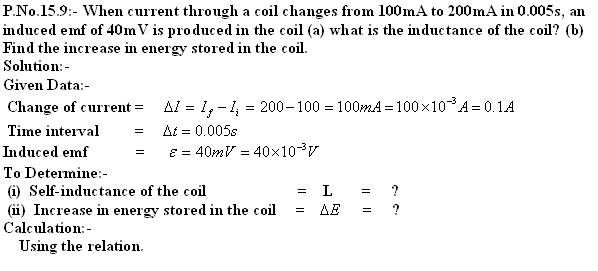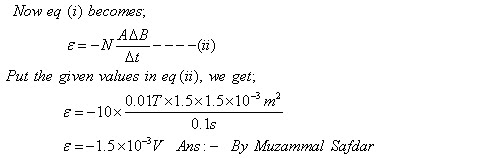P.No. 4.6:-
100m³ of water is pumped from a reservoir into a tank, 10m higher than the
reservoir, in 20 minutes. Find
(a) The increase in P.E
(b) The power delivered by the pump.
Solution:-
Given data:-
Volume of water = V = 100m³
Height = h = 10m
Time taken = t = 20min
= 20 × 60s = 1200s
To determine:-
Potential energy = P.E =?
Power = P =?
Calculation:-
( we know that work
done is equal to change in energy it may be kinetic or potential but in this case only P.E is involved. So,)
Work
done = Increase in P.E
= mgh
To
determine mass ‘m’ we first take
Density = mass/volume
Or, ρ = m/V